The Structure of Comb – Part 1
The Structure of Comb – Part 1
The Bee World – July, 1921 – Pages 37-38
By MISS ANNIE D. BETTS, B.Sc.
From references by the classical writers it is clear that the comb of the honey-bee has been admired from very early times. This is not surprising; for it would be difficult to find any human engineering achievement, even in modern aeronautical practice, that surpasses the honey-comb as a solution of the problem of combining light weight and great strength. Even if the ancients did not fully realise this, yet the beautiful regularity of the hexagonal network of cell-mouths could not fail to impress them; and it is not astonishing that the first known research on the structure of comb deals with the hexagonal form of the cells. Its author was Zenodorus, of Sicily, in the second century B.C., shortly after the time of Archimedes. Zenodorus proved that, of the three regular figures that will completely fill a plane surface (namely, the equilateral triangle, the square, and the regular hexagon), the hexagon has the greatest content for a given circumference. Pappus (ca. A.D. 500) copied from Zenodorus, and remarked that the bees wisely choose that one of the three forms for the cell-mouth which they suspect will contain most honey for the same expenditure of wax in its construction. This suggestion, that the bees economise wax, grew later into a wonderful myth, far removed from the realities of the matter.
The ideal form of the bee’s cell – seldom completely realised in actuality – is that of a regular six-sided prism, the base of which is formed of three rhombs of lozenges meeting in a point at the bottom of the cell (see Fig. 13).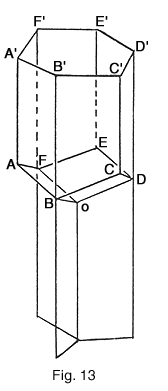
A’B’C’D’E’F’ is the cell-mouth; A’A, B’B, etc., are the edges of the cell; ABOF, CDOB, EFOD are the three rhombs; O being the bottom of the cell. Let us now consider the other side of the comb. From O there starts a cell-edge similar to those at A’A, C’C, or E’E; so that the three rhombs each form part of the base of a different cell on the other side of the comb; A, C, and E being the bottom points of these three cells, and correspending to O in the first cell. The edges B’B, D’D, and F’F are continuous right through the comb from one side to the other; a point that is probably of importance in counecton with the well-known and hitherto unexplained ”pitch” of the cells.
The plane passing through the points BDF is easily seen to lie in the exact middle of the comb (supposing the cells to be of equal depth on both sides); and if the pyramidal bases were replaced by flat ones in this plane, the cells would be unaltered in volume. Their surface would, however, be increased, as can be shown by a not very difficult calculation (here omitted to save space). The famous “problem of the bee’s cell,” around which the myth referred to grew up, is simply this: Find the shape of the rhombs, in a cell of the form shown in the diagram, such that the total area shall be a minimum, the other dimensions of the cell being unaltered. The answer gives us rhombs with angles respectively equal to 109 deg. 28′ 16.4″ and 70 deg. 31′ 43.6″. This result assumes that the walls and base-rhombs are all of equal thickness (which is not the case), that no wax is used to strengthen the edges of the cells (whereas about one-third of the total wax used goes for this purpose), and that all lines are straight and the cell quite regular (which is not so in actual comb). It will be seen, therefore, that this problem, however interesting to the mathematician, has but a slender connection with the bee’s cell as it really is. This did not trouble the various investigators who were jointly responsible for the myth, because they were either mathematicians with no knowledge of bees, or else naturalists with an equally profound ignorance of mathematics. Consequently, none of them was in a position adequately to criticise the others’ work:
The history of the subject is briefly this. After Pappus, no one seems to have studied the bee’s cell till Kepler, the astronomer, in 1611, published a very good description of it. He was apparently the first to notice the rhombs of the base, and was evidently quite familiar with bees at work.
The confusion begins in 1712, when Maraldi, an Italian astronomer, studied the cell, measured roughly the angles A’AB, A’AF, and BAF, and found them approximately equal to one another. He then calculated that, if these angles really were equal, they must each be of about 109 deg. 28′. Maraldi is an “awful warning” to us all to express ourselves quite clearly, so as to avoid all danger of being misunderstood. By using somewhat involved phraseology, he succeeded in conveying to the French naturalist Reaumur (some years later) the idea that he had found this value of 109 deg. 28′ by measurement! A feat which, as several writers have since remarked, was impossible with the instruments then in existence, even if the cells were regular, which they are not. Reaumur suspected that the bees economised wax, so asked a mathematical friend, Koenig, to work out the “problem of the bee’s cell” above referred to. Koenig did so, and gave the larger angle of the rhombs as 109 deg. 26′. Later investigations showed that 109 deg. 28′ was the correct answer (to the nearest minute) and that Koenig had made a slip in his arithmetic. Subsequently numerous foolish persons (prominent among whom was Lord Brougham) rushed in with triumphant observations to the effect that “the bee was right and the mathematician wrong,” and made much theological capital out of the fact(?) Actually, of course, it was Maraldi, not the bee, that was right; but nearly everyone followed Reaumur’s mistaken reading, and assumed that Maraldi had not calculated the angle, but had obtained it by measurement.
Many other investigators have studied the bee’s cell; some of them were careful to examine specimens of comb, and to note the irregularities of the cells, but many considered the problem only as one in pure mathematics, and their results need not be further discussed here. One of the best and latest papers on the subject is that by H. Vogt (Breslau. 1911). He gives an excellent account of the history of the problem (though the literature he consulted comprises only about one-third of the total in existence), and also of the results of his own measurements. These were made mostly on three or four combs sent him for the purpose, and on plaster-of-Paris casts of cells of these combs. One feels that a greater variety of combs must be measured (lengthy and wearisome as the work would be) before quite reliable results can be obtained; but for the present Vogt’s conclusions may be taken to be the best available. He shows that the edges of the cell, as well as the rim, are strengthened by wax deposited in the angles formed by each pair of walls. About one-third of the total amount of wax is thus employed. The form of the base is more pointed than it would be, were economy of wax the guiding factor in its construction. Allowing for the thickening of the edges, and for the differences in thickness between the base and the side-walls of the cell, Vogt states that, for greatest economy of wax, the larger angle of the rhombs should he about 116 deg.; actually this angle is (on the average) about 107 deg. Drone comb is in general more irregular than worker comb. The hexagonal network of the cell-mouths is nearer to perfect regularity than any other parts of the cell; this network, curiously enough, is more regular in drone than in worker comb. The rhombs are 1.59 times as thick as the side-walls in worker comb: for drone comb the figure is 1.58.


